Sequence Partitioning

Given a sequence of N ordered pairs of positive integers (A_i, B_i), you have to partition it into several contiguous parts. Let p be the number of these parts, whose boundaries are (l₁, r₁), (l₂, r₂), ... ,(l_p, r_p), which satisfy l_i = r_{i − 1} + 1, l_i ≤ r_i, l₁ = 1, r_p = n. The parts themselves also satisfy the following restrictions:

1. For any two pairs (A_p, B_p), (A_q, B_q), where (A_p, B_p) is belongs to the T_pth part and (A_q, B_q) the T_qth part. If T_p < T_q, then B_p > A_q.

2. Let M_i be the maximum A-component of elements in the ith part, say

   M_i = max{A_li, A_li+1, ..., A_ri}, 1 ≤ i ≤ p

   it is provided that

   

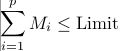
   where Limit is a given integer.

Let S_i be the sum of B-components of elements in the ith part. Now I want to minimize the value

max{S_i:1 ≤ i ≤ p}

Could you tell me the minimum?

The input contains exactly one test case. The first line of input contains two positive integers N (N ≤ 50000), Limit (Limit ≤ 2³¹-1). Then follow N lines each contains a positive integers pair (A, B). It's always guaranteed that

4 6
4 3
3 5
2 5
2 4

9